
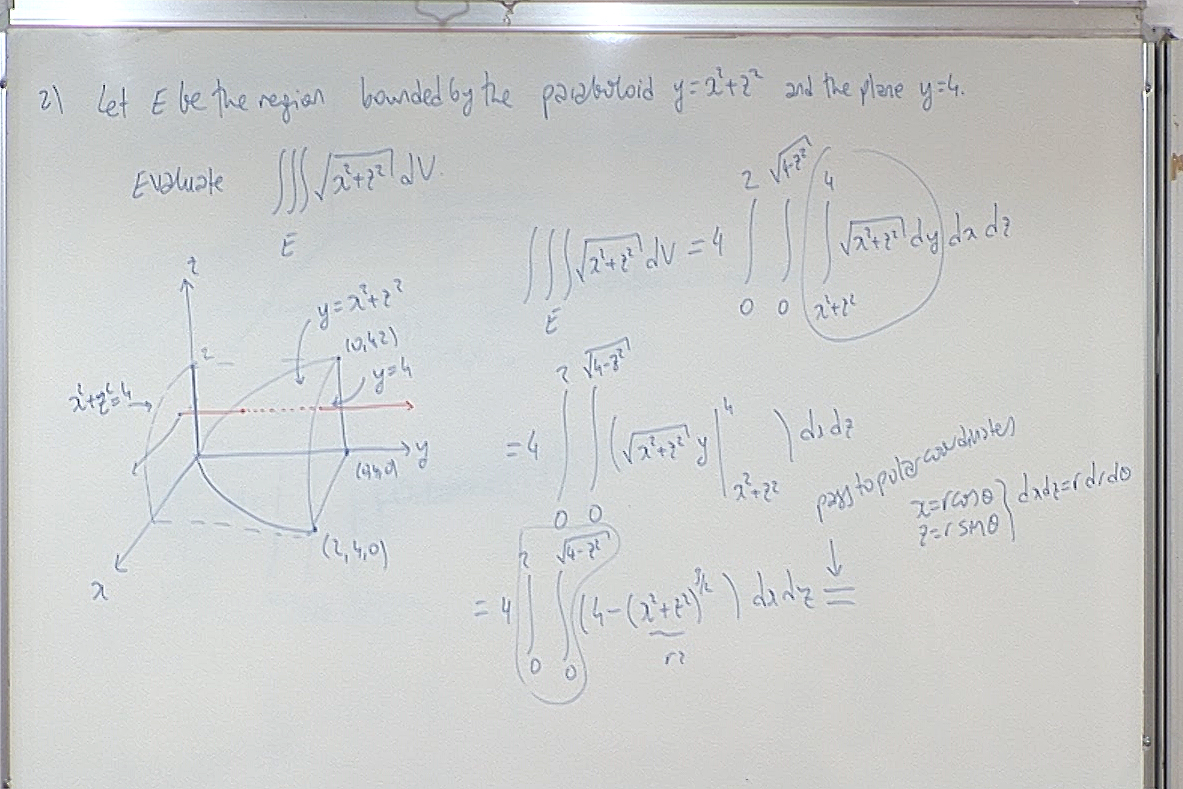
The last integral is wrong. The correct version and how it is to be continued is as follows. \[ =4\int_0^2 \int_0^{\sqrt{4-z^2}} \left[ 4\sqrt{x^2+z^2}-(x^2+z^2)^{3/2}\right] dxdz= 4\int_0^{\pi/2} \int_0^2 (4r-r^3)rdrd\theta=\frac{128}{15}\, \pi\approx 26.8. \]



At this point we had a quiz. Here is the question.

