227. Bilkent, 19 February 2010, Friday, 15:40
     Ali Sinan Sertöz-[Bilkent University]-Grothendieck-Riemann-Roch Theorem 
            

     Abstract:  We will conclude last term's seminar on K-theory with an application to algebraic geometry by developing Grothendieck's Riemann-Roch theorem. The talk will be expository and will be accessible even to those who do not remember much of last semester's talks!

      

228. ODTU, 26 February 2010 Friday, 15:40
     Deniz Kutluay-[Bilkent University]-Jones Polynomial
     
     

     Abstract: In 1984, V. Jones introduced a new (polynomial) knot invariant by using an operator algebra. Later, it became clear that this polynomial can be obtained by several different methods. We will pick a simple approach, namely defining it by means of the slightly different Kauffman bracket polynomial. We will then consider Jones polynomials of alternating links. In the remaining time, we will finish with the proofs of Tait's conjectures (due to K. Murasugi) by using Jones Polynomial.

      

229. Bilkent, 5 March 2010 Friday, 15:40
     Deniz Kutluay-[Bilkent University]-Tait's Conjectures
      

     Abstract: P.G. Tait conjectured, in 1898, that a reduced alternating diagram of a knot achieves the minimum possible number of crossings for that knot (1), and writhe of such diagrams of the same knot is the same (2). We will first give K. Murasugi's proof to (1) which involves usage of Jones polynomial. We will then use the idea of taking parallels of diagrams (due to R.A. Stong) to prove (2).

      

230. ODTU, 12 March 2010 Friday, 15:40
     İnan Türkmen-[Bilkent University]- Detecting Indecomposable Higher Chow Cycles
     
     

     Abstract: Spencer Bloch defined the higher Chow in mid 80's as a "natural" extension of classical Chow groups and analysed basic properties of these groups in terms of maps to Deligne Cohomology, named regulators. There is a subgroup of higher Chow groups, group of indecomposables, of special interest. In this talk I will introduce two different methods to detect indecomposables; regulator indecomposability and filtrations on arithmetic Hodge structures.

      

231. Bilkent, 19 March 2010 Friday, 15:40
     Alexander Degtyarev-[Bilkent University]- Dihedral covers of trigonal curves

      

     Abstract: We classify irreducible trigonal curves in Hirzebruch surfaces that admit a dihedral cover and study geometric properties of such curves. In particular, we prove an analog of Oka's conjecture stating that an irreducible trigonal curve admits an S_3 cover if and only if it is of torus type.

      

232. Bilkent, 26 March 2010 Friday, 15:40
     Bedia Akyar-[Dokuz Eylul University]- Prismatic sets in topology and geometry

      

     Abstract: We study prismatic sets analogously to simplicial sets except that realization involves prisms. In particular, I will mention the examples; the prismatic subdivision of a simplicial set S and the prismatic star of S. Both have the same homotopy type as S. Moreover, I will give the role of prismatic sets in lattice gauge theory, that is, for a Lie group G and a set of parallel transport functions defining the transition over faces of the simplices, we define a classifying map from the prismatic star to a prismatic version of the classifying space of G. In turn this defines a G-bundle over the prismatic star. This is a joint work with Johan L. Dupont.

      

      

233. ODTU, 9 April 2010 Friday, 15:40
     Yıldıray Ozan-[ODTU]- Algebraic K-theory in the study of regular maps in real algebraic geometry
     

      

     Abstract: After introducing some preliminary material about real algebraic varieties I will try to summarize how algebraic K-theory is used to study regular maps between real algebraic varieties. Namely, I will talk about the results of Loday and Bochnak-Kucharz which mainly show that regular maps between certain products of spherees are all null-homotopic. For example, Loday showed that any regular map from S1 x S1 to S2 is homotopically trivial, where Sk is the unit sphere in Rk+1.

      

234. ODTU, 16 April 2010 Friday, 15:40
     Ali Kemal Uncu-[TOBB ETU]-  Modular symbols on congruence subgroups of SL₂(Z)

      

     Abstract: The talk will be about finding the Fourier coefficients of a modular form of the given even weight on a congruence subgroup of SL2(Z). We will work with the Riemann surface related to the congruence subgroup of SL2(Z), define modular symbols and give the relation between modular symbols and Fourier coefficients of modular forms.

      

235. ODTU, 30 April 2010 Friday, 15:40
     Ergün Yalçın-[Bilkent University]-Koszul Resolutions and the Lie Algebra Cohomology 

      

     Abstract: Cohomology of a Lie algebra is defined both as the cohomology of its universal algebra and via a Koszul resolution. I will introduce both of the definitions and discuss their equivalence. Then, I will show how the Lie algebra cohomology appears in the integral cohomology calculation of a group extension.

      

236. Bilkent, 7 May 2010 Friday, 15:40
     Özgün Ünlü-[Bilkent University]- Homologically trivial group actions on products of spheres

      

     Abstract: In this talk, I will discuss some constructions of free group actions on products of spheres with trivial action on homology.

      

237. ODTU, 14 May 2010 Friday, 15:40
     Hamza Yeşilyurt-[Bilkent University]-Rogers-Ramanujan Functions

      

     Abstract:  We present several identities for the Rogers-Ramanujan functions along with their partition theoretic interpretations and conclude with our recent work on such identities.

 

 

238. Bilkent, 28 May 2010 Friday, 15:40
     Mutsuo Oka-[Tokyo University of Science]-Polar weighted homogeneous polynomials and mixed Brieskorn singularity

      

     Abstract: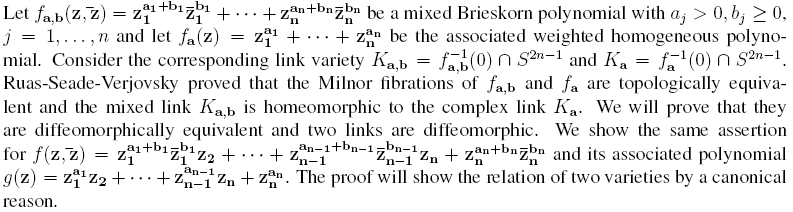

2010 Fall Talks

 

239. Bilkent, 1 October 2010, Friday, 15:40
     
     Alexander Degtyarev-[Bilkent University] - The Alexander module of a trigonal curve
            

     Abstract:  The Alexander module of an algebraic curve is a certain purely algebraic  invariant of the fundamental group of (the complement of) the curve. Introduced by Zariski and developed by Libgober, it is still a subject of intensive research. We will describe the Alexander modules and Alexander polynomials (both over  Q and over finite fields Fp ) of a special class of curves, the so called generalized trigonal curves.  The rational case is closed completely; in the case of characteristic p>0, a few points remain open. (Conjecturally, all polynomials that can appear are indeed listed.) Unlike most known divisibility theorems, which rely upon the degree and the types of the singularities of the curve, our bounds are universal: essentially, the Alexander module of a trigonal curve can take but a finitely many values.

      

240. ODTU, 8 October 2010 Friday, 15:40
     
     Ömer Küçüksakallı-[ODTU] - Frey Curves and Fermat's Last theorem
     
     

     Abstract: The curious history of Fermat's Last Theorem starts with Fermat's famous marginal commentary. The quest for the solution of this problem has created theories which affect all of mathematics. In this seminar, we will talk about Ribet's theorem which states that modularity theorem (previously known as Taniyama-Shimura conjecture) implies Fermat's Last Theorem. A central role in Ribet's proof is played by elliptic curves introduced by Frey.

      
     -----------------------------------------------------------------------------------------
     On 13-15 October, we are having an Algebra and Number Theory Symposium in 
     honor of Prof Mehpare Bilhan's retirement. 
     There will be no Algebraic Geometry talk this week. 
     -----------------------------------------------------------------------------------------
     

241. Bilkent, 22 October 2010 Friday, 15:40
     
     Christophe Eyral-[Aarhus University] - A short introduction to Lefschetz theory on the topology of algebraic varieties
     
     

     Abstract:

     -----------------------------------------------------------------------------------------------------------------------
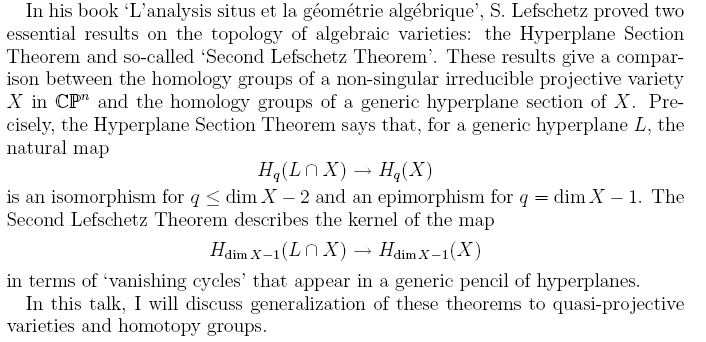
     29 October is Republic Day, a national day for Turkey. No talks!
     ------------------------------------------------------------------------------------------------------------------------
     

242. Bilkent, 5 November 2010 Friday, 16:00
     
     Muhammed Uludağ-[Galatasaray University] - The Groupoid of Orientation Twists

      

     Abstract: This is an essay to define a higher modular groupoid. The usual modular groupoid of triangulation flips admits ideal triangulations of surfaces of fixed genus and punctures as objects and flips as morphisms. The higher groupoid of orientation twists admits usual modular groupoids as its objects.

      

243. ODTU, 12 November 2010 Friday, 15:40
     
     İnan Utku Türkmen-[Bilkent University] - An Indecomposable Cycle on Self Product of Sufficiently General 
     Product of Two Elliptic Curves
     
     

     Abstract: The group of indecomposables is too complicated to compute in general and the results in literature are cenrered around proving that this group is non-trivial or in certain cases finitely generated. In this talk I will focus on the group of indeconposables of self product of sufficiently general product of two elliptic curves, namely; CH3ind(E1x E2 x E1 x E2). I will review the results in literature related with this group and sketch an alternative proof for non-triviality of this group using a constructive method.

     -----------------------------------------------------------------------------------------------------------------------
     16-19 November is a religious holiday in Turkey. No talks!
     ------------------------------------------------------------------------------------------------------------------------

      

244. ODTU, 26 November 2010 Friday, 15:40
     
     Mehmetcik Pamuk-[ODTU] - s-cobordism classification of 4-manifolds

      

     Abstract: In this talk we are going to show how one can use the group of homotopy self-equivalences of a 4-manifold together with the modified surgery of Matthias Kreck to give an s-cobordism classification of topological 4-manifolds. We will work with certain fundamental groups and give s-cobordism classification in terms of standard invariants.

      

245. Bilkent, 3 December 2010 Friday, 15:40
     
     Ergün Yalçın-[Bilkent University] - Productive elements in group cohomology

      

     Abstract:  I will give the definition of a productive element in group cohomology and describe a new approach to productive elements using Dold's Postnikov decomposition theory for projective chain complexes. The motivation for studying productive elements comes from multiple complexes which is an important construction for studying varieties of modules in modular representation theory.

      

246. ODTU, 10 December 2010 Friday, 15:40
     
     Mustafa Kalafat-[University of Wisconsin at Madison and ODTU] - 
     Hyperkahler manifolds with circle actions and the Gibbons-Hawking Ansatz
     
     

     Abstract: We show that a complete simply-connected hyperkahlerian 4-manifold with an isometric triholomorphic circle action is obtained from the Gibbons-Hawking ansatz with some suitable harmonic function.

     
     
     

247. Bilkent, 17 December 2010 Friday, 15:40
     
     Kürşat Aker-[Feza Gürsey] - Multiplicative Generators for the Hecke ring of the Gelfand Pair (S(2n), H(n))

      

     Abstract: For a given positive integer n, Gelfand pair (S(2n), H(n)) resembles the symmetric group S(n) in numerous ways. Here, H(n) is a hyperoctahedral subgroup of the symmetric group S(2n). In this talk, we will exhibit a new similarity between the Hecke ring of the pair (S(2n), H(n)) and the center of the integral group ring of S(n).   Multiplicative generators for centers of integral symmetric groups were first identified by Farahat and Higman, which were later shown to be elementary symmetric polynomials in the celebrated Young-Jucys-Murphy elements by Jucys.  In this talk, we will present a set of multiplicative generators for the Hecke ring of (S(2n), H(n)), affirming a conjecture of Matsumoto, who showed these elements are elementary symmetric polynomials evaluated at odd Young-Jucys-Murphy elements after projecting from the integral group ring of S(2n) to the Hecke ring of (S(2n),H(n)). This is a joint work with Mahir Bilen Can, Tulane University.  * Reference: http://front.math.ucdavis.edu/1009.5373

     
     
     

248. ODTU, 24 December 2010 Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent] - Counting the number of lines on algebraic surfaces 

      

     Abstract:   This is mostly an expository talk on the problem of counting the number of lines on an algebraic surface. The problem is to respect the rigidity of the line as opposed to accepting all rational curves as lines. Surprisingly some of the work done by Segre has not yet been matched by contemporary techniques. We will summarize what is known and speculate about what can be known!

-----------------------------------------------------------------------------------------------------------------------
31 December  afternoon is no time to hold seminars on this planet! No talks!
------------------------------------------------------------------------------------------------------------------------


 

 

2011 Spring Talks

 

261. Bilkent, 7 October  2011, Friday, 15:40
     
     Alexander Degtyarev-[Bilkent University] - Products of two Dehn twists and real Lefschetz fibrations
     
     

     Abstract:  (joint with Nermin Salepci, Université de Lyon)  An object repeatedly occurring in algebraic geometry is a fibration with singular fibers. If the base is a topological disk and the number of singular fibers is finite, the topology of such a fibration can adequately be described by the so called monodromy factorization of the monodromy at infinity (boundary of the disk), regarded up to a certain collection of moves, called Hurwitz moves and, possibly, global conjugation. We consider the simplest nontrivial case: factorizations into product of two Artin generators in the braid group B_3 (equivalently, two Dehn twists in the mapping class group of a torus). Even here, the results obtained are quite unexpected; considering the known examples, the general case (even in B_3) is very far from its complete understanding.    Trivial as it seems, this simplest case has a number of geometric applications. As a first one, we prove that any maximal real elliptic Lefschetz fibration over the sphere is algebraic. Other applications include the semi-simplicity statement for real trigonal M-curves in Hirzebruch surfaces. (One may try to speculate that products of two Dehn twists are still `tame' precisely because they are related to maximal geometric objects.)   The principal tool is a description of subgroups of the modular group in terms of a certain class of Grothendieck's dessins d'enfants, followed by high school geometry.

      

262. ODTU, 14 October  2011, Friday, 15:40
     
     Alexander Degtyarev-[Bilkent University] - Lines on quartic surfaces 
            

     Abstract:   The purpose of this expository talk is to lay a basis for Sinan's forthcoming account of our joint project. Recall that a quartic surface in P3 is merely a K3-surface equipped with a polarization of degree 4. Thus, I will give a gentle introduction to theory of K3-surfaces: the period space, the global Torelli theorem and surjectivity of the period map, and the implications of the Riemann--Roch theorem. I will explain how the problem of counting lines on a quartic can be reduced to a purely arithmetical question and, should time permit, give a brief account of the results obtained so far, viz. a more or less explicit description of the Picard group of the champion quartic.

     
     

263. Bilkent, 21 October  2011, Friday, 15:40
     
     Richard Gonzales-[Boğazici University] - KM theory of rationally smooth group embeddings. 
            

     Abstract:  Let G be a reductive group. A GxG-variety X is called an embedding of G if X is normal, projective, and contains G as an open dense orbit. Regular compactifications and standard embeddings are the main source of examples. In the former case, they are smooth varieties, and their equivariant cohomology has been explicitely described by Brion using GKM theory. His description relies on the associated torus embedding and the structure of the GxG-orbits. In contrast, standard embeddings constitute a much larger class of embeddings than the smooth ones, and their equivariant cohomology was, just until recently, only understood in some cases. Based on results of Renner, standard embeddings were known to come equipped with a canonical cell decomposition, given in terms of underlying monoid data.  The purpose of this talk is three-fold. First, I will give an overview of the theory of group embeddings, putting more emphasis on Renner's approach, and describe the structure of the so called rational cells. Secondly, I will explain how such cellular decompositions lead to a further application of GKM theory to the study of standard embeddings. Finally, I provide a complete description of the equivariant cohomology of any rationally smooth standard embedding. The major results of this talk are part of the speaker's PhD thesis.   References: Brion, M. ''The behaviour at infinity of the Bruhat decomposition''. Comment. Math. Helv. 73, pp. 137-174 (1998). Gonzales, R. ''GKM theory of rationally smooth group embeddings''. PhD thesis (2011).http://ir.lib.uwo.ca/etd/216/  Goresky, M., Kottwitz, R., MacPherson, R. ''Equivariant Cohomology, Koszul duality, and the localization theorem''. Invent. math. 131, pp. 25-83 (1998). Renner, L. '' The H-polynomial of an Irreducible Representation''. Journal of Algebra 332, pp. 159-186 (2011).  PS: The speaker is supported under TUBITAK ISBAP Grant  107T897 -Matematik İşbirliği Ağı: Cebir ve Uygulamaları.

     
     The afternoon of 28 October is a National Holiday.

     
     

264. Bilkent, 4 November  2011, Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent University] - An overview of counting lines on algebraic surfaces 
            

     Abstract:  I will wrap up my recent investigations on lines on surfaces with a view towards settling some problems jointly with Degtyarev.

     
     There is no talk on 11 November 2011 due to Kurban Bayramı.
     
     

265. ODTU, 18 November  2011, Friday, 15:40
     
     Mehmetcik Pamuk-[ODTU] - Surgery Method of Classifying Manifolds 
            

     Abstract:  The surgery method of classifying manifolds seeks to answer the following question: Given a homotopy equivalence of m-dimensional manifolds f: M -->  N, is f homotopic to a diffeomorphism ? The surgery theory developed by Browder, Novikov, Sullivan and Wall in the 1960’s provides a systematic solution to this problem.  My talk will aim to be a friendly introduction to the basic concepts of the surgery theory.

     
     

266. Bilkent, 25 November  2011, Friday, 15:40
     
     Selma A. Bhupal-[Hacettepe University] - On Piecewise Polynomial Functions and their Dimension 
            

     Abstract:  Splines or piecewise polynomial functions are used most commonly to approximate functions, especially by numerical analysts for approximating solutions to differential equation. Most recently, splines have also played an important role in computer graphics.  That’s why  it is of interest to study spline spaces. In this talk, we will discuss analyzing the piecewise functions with a specified degree of smoothness  on polyhedral subdivision of region on  Rn  and their dimension.

     
     

267. ODTU, 2 December  2011, Friday, 15:40
     
     Ahmet Beyaz-[ODTU] - Genus Zero Gromov-Witten Invariants 
            

     Abstract:  In this talk, we review the genus zero Gromov-Witten invariants by first defining them in a brief way and then applying them in examples of dimension four and six. We also prove that the use of genus zero Gromov-Witten invariants to distinguish the symplectic structures on a smooth 6-manifold is restricted in a certain sense.

     
     

268. Bilkent, 9 December  2011, Friday, 15:40
     
     Mustafa Kalafat-[ODTU] - Geometric Invariant Theory and Einstein-Weyl Geometry
           

     Abstract:  We give a survey of Geometric Invariant Theory for Toric Varieties, and present an application to the Einstein-Weyl Geometry. We compute the image of the Minitwistor space of the Honda metrics as a categorical quotient according to the most efficient linearization. The result is the complex weighted projective space CP_(1,1,2). We also find and classify all possible quotients.

     
     

269. ODTU, 16 December   2011, Friday, 15:40
     
     Ferruh Özbudak-[ODTU] - Finite number of Kummer cover and curves with many points 
            

     Abstract:  We study the fibre products of a finite number of Kummer covers of the projective line over finite fields. We determine the number of rational points of the fibre product under certain conditions. We also construct expicit examples of fibre products of Kummer covers with many rational rational points, which includes a record and two new entries of the current table of the ``manypoints". This is a report on a joint work with Burcu Gulmez Temur.

     
     

270. Bilkent, 23 December  2011, Friday, 15:40
     
     Mesut Şahin-[Karatekin University] - On Toric Codes 
            

     Abstract:  Toric codes are some evaluation codes obtained by projective toric varieties corresponding to convex lattice polytopes. We will explain how their basic parameters are related to the torus and the number of lattice points of the polytope and introduce certain generalizations. We will also review some recent results about the minimum distance.

     
     

271. ODTU, 30 December  2011, Friday, 15:40
     
     Alexander Klyachko-[Bilkent University] - Toric and Flag varieties 
            

     Abstract:  In the talk I will discuss the structure of toric variety XG equal to closure of a generic orbit of a maximal torus of a simple group G in its flag variety FG, the respective restriction map H*(FG)-->H*(XG) together with some applications.

      

272. Bilkent, 6 January  2012, Friday, 15:40
     
     Çetin Ürtiş-[TOBB] - Sphere Packings, Lattices and Theta Functions
       

     Abstract:  How should greengrocers most efficiently stack their oranges? How about pennies on a tabletop or atoms of a single element in a crystal? More than 400 years ago Kepler conjectured that the most efficient way is the face-centered cubic packing which is well known for greengrocers nowadays. Just recently a "proof" (referees are 99% are certain) for Kepler's conjecture is given. In this talk we will give a brief history of the conjecture and related problems. By considering the problem in higher dimensions we will illustrate some special cases and their applications to different areas of mathematics. In particular, the connection between lattices and theta functions will be discussed.

      

2012 Spring Talks

273. Bilkent, 2 March  2012, Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent University] - Atiyah-Singer Index Theorem - Preliminaries 
            

     Abstract:  Preliminaries will be discussed; mostly characteristic classes.

      

      

274. ODTU, 9 March  2012, Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent University] - Atiyah-Singer Index Theorem - Motivation and Statement 
            

     Abstract:  I will talk about the motivation for the index theorem and discuss the individual terms in the statement of the theorem.

     
     
     

275. Bilkent, 16 March  2012, Friday, 15:40
     
     İnan Utku Türkmen-[Bilkent University] - The de Rham and Dolbeault operators 
            

     Abstract:  We examine the consequences of applying the Atiyah-Singer Index Theorem to de Rham and Dolbeault operators.

     
     

276. ODTU, 23 March  2012, Friday, 15:40
     
     Mustafa Kalafat-[ODTU] - The Hodge operator 
            

     Abstract:  In this talk we will demonstrate that the application of the Atiyah-Singer Index Theorem to Hodge operator yields the Hirzebruch signature theorem.

     
     

277. Bilkent, 30 March   2012, Friday, 15:40
     
     Yıldıray Ozan-[ODTU] - The Dirac operator 
            

     Abstract:  In this talk we will discuss the application of the Atiyah-Singer Index Theorem to Dirac operator.

     
     

278. ODTU, 6 April  2012, Friday, 15:40
     
     Turgut Onder-[ODTU] - The ring K(X)
           

     Abstract:  In this talk we give a brief description of the ring K(X) of  stable vector bundles over X.

     
     

279. Bilkent, 13 April   2012, Friday, 15:40
     
     Turgut Onder-[ODTU] - The ring K(X), II 
            

     Abstract:  In this talk we continue to give a brief description of the ring K(X) of  stable vector bundles over X.

     
     

280. Bilkent, 20 April  2012, Friday, 15:40
     
     Asli Guclukan Ilhan-[Bilkent] - The topological index B 
            

     Abstract:  In this talk we will elaborate on the topological index B as covered in Shanahan's boook.

     
     

281. ODTU, 27 April  2012, Friday, 15:40
     
     Mehmetcik Pamuk-[ODTU] - Pseudodifferential operators 
         

     Abstract:  In this talk we will discuss pseudodifferential operators and their suitable generalizations as discussed in Shanahan's book.

      

282. Bilkent, 4 May  2012, Friday, 15:40
     
     Özgün Ünlü-[Bilkent] - Construction of the index homomorphism
       

     Abstract:  In this talk we will discuss the construction of the index homomorphism as given in Shanahan's book.

      

283. Bilkent, 11 May  2012, Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent University] - Proof of the index theorem 
            

     Abstract:  In this talk we will discuss the main ideas surrounding the proof of the index theorem as given in Shanahan's book.

      

2012 Fall Talks

284. Bilkent, 21 September  2012, Friday, 15:40
     
     Alexander Degtyarev-[Bilkent] - On lines on smooth quartics
            

     Abstract:  (a never ending joint project with I. Itenberg and S. Sertoz) It is a common understanding that, thanks to the global Torelli theorem and the surjectivity of the period map, any reasonable question concerning the topology and geometry of $K3$-surfaces can be reduced to a certain arithmetical problem. We tried to apply this ideology to the study of the possible configurations of straight lines on a nonsingular quartic surface in $ \mathbb{P}^3$. According to C. Segre, a nonsingular quartic in $\mathbb{P}^3$ may contain at most 64 lines, and one explicit example of a surface with exactly 64 lines is known. The original proof, using classical algebraic geometry in the Italian school style, is very complicated. We managed to reprove Segre's result using the contemporary arithmetical approach. In addition, we prove that, up to projective equivalence, a nonsingular quartic with 64 lines is unique. Furthermore, we show that a real nonsingular quartic may contain at most 56 real lines and, conjecturally, such a quartic is also unique (although the latter statement is not quite definite yet).  Alas, the proof is transparent but heavily computer aided, the principal achievement being a stage at which my laptop can handle it in finite time (although a human still cannot).

      

      

285. ODTU, 28 September   2012, Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent] - The Chow ring of $\mathbb{G}(1,3)$
            

     Abstract:  I will start with Chapter 2 of Eisenbud-Harris notes and after a brief introduction I will describe the Chow ring of $\mathbb{G}(1,3)$, with a view toward counting the number of lines which meet four general lines in $\mathbb{P}^3$.

     
     
     

286. Bilkent, 5 October  2012, Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent]  - The Chow ring of $\mathbb{G}(1,3)$, part II
            

     Abstract:  I will continue to explore the geometry of Grassmannians, after which I will start discussing the Chow ring of $\mathbb{G}(1,3)$. I hope to have time to talk about the number of lines meeting four general lines in space.

     
     

287. ODTU, 12 October  2012, Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent]  - The Chow ring of $\mathbb{G}(1,3)$, part III
            

     Abstract:  I will  start by describing the Chow ring of  $\mathbb{G}(1,3)$ and then attack the "Keynote Questions" quoted at the beginning of the chapter.

     
     

288. Bilkent, 19 October   2012, Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent]  - The Chow ring of $\mathbb{G}(1,3)$, part IV-last!
            

     Abstract:  I will complete the multiplication table of the Chow ring of  $\mathbb{G}(1,3)$ and then attack the "Keynote Questions" quoted at the beginning of the chapter. Rain or shine, I will finish my talk series this week!

     
     

289. ODTU, 2 November  2012, Friday, 15:40
     
     Tolga Karayayla-[ODTU] - Schubert calculus, Chow ring of Grassmannians, part I
           

     Abstract: We generalize the discussion on the intersection theory on G(1,3) given in the previous seminars to the Grassmanian variety G(k,n). We are going to define the Schubert cells and cycles and discuss their properties known as Schubert Calculus. We will determine the Chow Ring A(G(k,n)) and mention some applications to intersection theory problems.

     
     

290. Bilkent, 9 November  2012, Friday, 15:40
     
     Tolga Karayayla-[ODTU] - Schubert calculus, Chow ring of Grassmannians, part II
            

     Abstract:  We generalize the discussion on the intersection theory on G(1,3) given in the previous seminars to the Grassmanian variety G(k,n). We are going to define the Schubert cells and cycles and discuss their properties known as Schubert Calculus. We will determine the Chow Ring A(G(k,n)) and mention some applications to intersection theory problems.

     
     

291. ODTU, 16 November  2012, Friday, 15:40
     
     Müfit Sezer-[Bilkent] - Invariants of the Klein four group in chracteristic two
            

     Abstract:  We consider an indecomposable representation of the Klein four group over a field of characteristic two and compute a generating set for the corresponding invariant ring up to a localization. We also obtain a homogeneous system of parameters consisting of twisted norms and show that the ideal generated by positive degree invariants is a complete intersection. (joint with J. Shank)

     
     

292. Bılkent, 23 November  2012, Friday, 15:40
     
     Emre Şen-[Bilkent] - Chow Groups of Rational Equivalence Classes of Cycles
         

     Abstract:  First we start with defining rational equivalence between two cycles. Then we define the chow group as a group of rational equivalence classes. Then we will present essential theorems and propositions which are developed at the fourth chapter (D. Eisenbud and J. Harris, All That Intersection Theory in Algebraic Geometry) to solve the keynote question  b:  "Let $L,Q\subset \mathbb{P}^3$ be a line and a nonsingular conic in $ \mathbb{P}^3$. Is $\left( \mathbb{P}^3\setminus L\right)\cong\left( \mathbb{P}^3\setminus Q\right)$ as schemes?" (ref. page 139)

      

293. Bilkent, 30 November  2012, Friday, 15:40
     
     Özgür Kişisel-[ODTU] - Tropical Intersections
       

     Abstract:  After an introductory discussion of tropical varieties, I intend to talk about tropical intersections and in particular the tropical Grassmannian.

     
     7 December 2012, Friday
     This week's seminar is cancelled due to the traffic of Docent juries taking place this week.
            
     

294. ODTU, 14 December  2012, Friday, 15:40
     
     Tolga Karayayla-[ODTU] - Schubert calculus, Chow ring of Grassmannians, part III
            

     Abstract:  We generalize the discussion on the intersection theory on G(1,3) given in the previous seminars to the Grassmanian variety G(k,n). We are going to define the Schubert cells and cycles and discuss their properties known as Schubert Calculus. We will determine the Chow Ring A(G(k,n)) and mention some applications to intersection theory problems.

      

295. ODTU, 21 December  2012, Friday, 15:40
     
     Nil Şahin-[ODTU] - Singularity Theory and Arf Rings
            

     Abstract:  Arf Closure of a local ring corresponding to a curve branch, which carries a lot of information about the branch, is an important object of study, and both Arf rings and Arf semigroups are being studied by many mathematicians, but there is not an implementable fast algorithm for constructing the Arf closure. The main aim of this work is to give an easily implementable fast algorithm for constructing the Arf closure of a given local ring. The speed of the algorithm is a result of the fact that the algorithm avoids computing the semigroup of the local ring. Moreover, in doing this, we give a bound for the conductor of the semigroup of the Arf Closure without computing the Arf Closure by using the theory of plane branches. We also give an exposition of plane algebroid curves and present the SINGULAR library written by us to compute the invariants of plane algebroid curves.

      

296. Bilkent, 28 December  2012, Friday, 15:40
     
     Mustafa Kalafat-[Tunceli] - Einstein-Hermitian 4-Manifolds of Positive Bisectional Curvature
            

     Abstract:  We show that a compact complex surface together with an Einstein-Hermitian metric of positive holomorphic bisectional curvature is biholomorphically isometric to the complex projective plane with its Fubini-Study metric up to rescaling.  (Joint work with C.Koca.)

      

2013 Spring Talks

297. ODTU, 1 March 2013, Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent] - Lines on hypersurfaces
            

     Abstract:  This is going to be an informal talk on the dimension of the Fano variety of $k$-linear subspaces of projective hypersurfaces, with emphasis on the $k=1$ case. I will losely follow the contents of Chapter 7 and 8 of Eisenbud and Harris' to-be-published book Intersection Theory in Algebraic Geometry.

     
     

298. Bilkent, 8 March 2013, Friday, 15:40
     
     Ali Sinan Sertöz-[Bilkent] - Chern classes as degeneracy cycles and some applications
            

     Abstract:  We continue our leisurely paced learning seminar on Eisenbud and Harris' notes. I will start by reminding the definition of Chern classes as degeneracy cycles and continue with  the calculation of the Chern classes of some interesting bundles. As an application I will talk about how these approaches are used to come up with the number 27, the number of  lines on a smooth cubic surface in $\mathbb{P}^3$. Time permitting, I will also attempt to explain solutions to some of the keynote questions posed at the beginning of chapter 8.

     
     

299. ODTU, 15 March 2013, Friday, 15:40
     
     Sergey Finashin-[METU] - Invariants of complex algebraic surfaces via differential topology
           

     Abstract: Discovery of the gauge-theoretic invariants (Donaldson's and later Seiberg-Witten's) brought a number of fundamental discoveries completely changing the landscape of Low-dimensional topology. I will review essentials of this theory tracing its later development (Ozsvath-Szabo theory) and focusing on the applications to algebraic geometry.

     
     

300. Bilkent, 22 March 2013, Friday, 15:40
     
     Sergey Finashin-[METU] - Invariants of complex algebraic surfaces via differential topology, II
            

     Abstract:  After giving a general definition of Seiberg-Whitten invariants, their meaning in the case of Kahler surfaces will be explained. Some applications and developments will be discussed.

     
     

301. ODTU, 29 March 2013, Friday, 15:40
     
     Yıldıray Ozan-[METU] - Some applications of Differential Topological Invariants to Algebraic Surfaces
            

     Abstract:  After a short review of differential topological invariants of smooth manifolds, we will discuss some applications to algebraic surfaces. As an example I will discuss the complete intersection surfaces, presented by W. Ebeling (Invent. 1990), which form a pair of nondiffeomorphic but homeomorphic surfaces.

     
     

302. Bılkent, 5 April 2013, Friday, 15:40
     
     Özgür Ceyhan-[Luxembourg] - Feynman integrals as periods in configuration spaces
     
     (This talk is organized in collaboration with Bilkent Department of Mathematics.)
         

     Abstract:  Mid 90's, Broadhurst and Kreimer observed that multiple zeta values persist to appear in Feynman integral computations. Following this observation, Kontsevich proposed a conceptual explanation, that is, the loci of divergence in these integrals must be mixed Tate motives. In 2000, Belkale and Brosnan disproved this conjecture. In this talk, I will describe a way to correct Kontsevich's proposal and show that the regularized Feynman integrals in position space setting as well as their ambiguities are given in terms of periods of suitable configuration spaces, which are mixed Tate. Therefore, the integrals that are of our interest are indeed $\mathbb{Q}[1/2 \pi i]$-linear combinations of multiple zeta values. This talk is based on a joint work with M. Marcolli.

      

303. ODTU, 12 April 2013, Friday, 15:40
     
     Emre Coşkun-[METU] - An Introduction to Moduli Problems 
       

     Abstract:  In this two-part talk, we will define a moduli problem, and we will discuss the solutions in a number of well-known cases. We start by defining the moduli functor. Next, we show that the Grassmannian functor is represented by the Grassmann variety of linear subspaces of projective space. After discussing the Quot scheme in very general terms, we move to the construction of the moduli space of vector bundles of given rank and degree on an algebraic curve.

     
            

304. Bilkent, 19 April 2013, Friday, 15:40
     
     Emre Coşkun-[METU] - An Introduction to Moduli Problems, II
            

     Abstract:  In this two-part talk, we will define a moduli problem, and we will discuss the solutions in a number of well-known cases. We start by defining the moduli functor. Next, we show that the Grassmannian functor is represented by the Grassmann variety of linear subspaces of projective space. After discussing the Quot scheme in very general terms, we move to the construction of the moduli space of vector bundles of given rank and degree on an algebraic curve.

      

305. ODTU, 26 April 2013, Friday, 15:40
     
     Koray Karabina-[Bilkent] - Elliptic Curve Discrete Logarithm Problem
            

     Abstract:  Let $G=\langle g \rangle$ be a finite group generated by $g$. Given $h\in G$, the discrete logarithm problem (DLP) in $G$ with respect to the base $g$ is computing an integer $a$ such that $h=g^a$. The security of many cryptographic protocols relies on the intractability of DLP in the underlying group. Pollard's rho method is a general purpose algorithm to solve DLP in finite groups, and runs in fully-exponential expected time of $\sqrt{|G|}$. Some special purpose algorithms, such as index calculus method, can solve DLP in finite field groups in sub-exponential time. The lack of an efficient DLP solver for elliptic curve groups has been the main reason for elliptic curve based cryptography to shine compared to finite field based cryptography and the RSA cryptosystem. Recent results show that index calculus can be modified to solve ECDLP in certain settings faster than Pollard's rho algorithm. I will discuss recent developments in using index calculus method to solve ECDLP, and some restrictions of the method that motivate many open problems in the area.

      

306. Bilkent, 3 May 2013, Friday, 15:40
     
     Alexander Degtyarev-[Bilkent] - On the Bertini involution
            

     Abstract:  Paraphrasing A. Marin, we are "à la recherche de la géométrie algébrique perdue": a journey to forgotten algebraic geometry. Following Ethel I. Moody and taking her notes a bit further, I will discuss explicit equations  (not just a formal construction in terms of  some sheaves and their sections) describing the beautiful Bertini involution and related maps and curves. Should time permit, I will also say a few words justifying my interest in the subject: the Bertini involution can be used to produce explicit equations of the so-called maximizing plane sextics. In theory, all sextics that are still not understood can be handled in this way, but alas, sometimes Maple runs out of memory trying to solve the equations involved.

      

      

307. ODTU, 10 May 2013, Friday, 15:40
     
     Mustafa Kalafat-[Tunceli] - Topology of $G_2$ manifolds
            

     Abstract:  We analyze the topological invariants of some specific Grassmannians, the Lie group $G_2$, and give some applications. This is a joint work with Selman Akbulut.

     
     
     

308. Bilkent, 17 May 2013, Friday, 15:40  
     
     Emre Can Sertöz-[Humboldt] - Idea of the Moduli Space of Curves
        

     Abstract:  By considering Riemann surfaces from several different angles, we will see that there are many seemingly different ways to vary the complex structure on a surface, getting different Riemann surfaces. So we can ask "What is the most natural way to vary Riemann Surfaces?". This is what the moduli space construction answers, and we will talk about it. Also we will see why we need some extra structure on the moduli space besides the classical structures that come via a manifold (or a scheme).

2013 Fall Talks

309. ODTU, 4 October 2013, Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Affine toric varieties, cones, lattices, semigroup rings, toric ideals
            

     Abstract:  In this talk, we give the classical definition of a toric variety  involving the torus action and provide examples to illustrate it.  We introduce two important lattices that play important roles in  the theory of algebraic tori and demonstrate how they arise  naturally in the toric case. Finally, we introduce affine toric  varieties determined by strongly convex rational cones.

     
     

310. Bilkent, 11 October 2013, Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Fans, toric variety of a fan via gluing affine toric varieties, Orbit-Cone correspondence
            

     Abstract:  In this talk, we introduce fans and the (abstract) toric variety determined by a fan via gluing affine toric varieties defined by the cones in the fan. We include some examples and conclude with the correspondence between orbits of the torus action and the cones in the fan.

     
     18 October is Kurban Bayramı.
     
     

311. ODTU, 25 October 2013, Friday, 15:40
     
     Mustafa Kalafat-[Tunceli] - Examples. Blow ups. Resolution of Singularities. Torus action. Orbits. Divisors.
           

     Abstract: We will revise the material on toric varieties with emphasis on examples and introduce some new concepts as time permits.

     
     

312. Bilkent, 1 November 2013, Friday, 15:40
     
     Mustafa Kalafat-[Tunceli]- Blow ups. Resolution of Singularities. Torus action. Orbits. Divisors - I
            

     Abstract:  We will continue to discuss the material in Brasselet's exposition  "Geometry of toric varieties", sections 5 and 6, as time permits.

     
     

313. ODTU, 8 November 2013, Friday, 15:40
     
     Mustafa Kalafat-[Tunceli]- Blow ups. Resolution of Singularities. Torus action. Orbits. Divisors - II
            

     Abstract:  We will complete our discussion of the material in Brasselet's exposition  "Geometry of toric varieties", sections 5 and 6.

     
     

314. Bılkent, 15 November 2013, Friday, 15:40
     
     Mustafa Kalafat-[Tunceli]- Blow ups. Resolution of Singularities. Torus action. Orbits. Divisors - III
         

     Abstract:  We will complete our discussion with more examples.

      

     21-24 Nov 2013 Japanese Turkish Joint Geometry Meeting, Galatasaray University, İstanbul
            

315. ODTU, 29 November 2013, Friday, 15:40
     
     Alexander Degtyarev-[Bilkent] - Resolutions of singularities, Viro’s patchworking, and tropical geometry
            

     Abstract:  In this very introductory talk I will try to discuss the interplay between such concepts as embedded toric resolutions of singularities via Newton polygons, Viro’s combinatorial patchworking, and tropical geometry.

      

316. Bilkent, 6 December 2013, Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Projective toric varieties
            

     Abstract: We start with the definition of normal, very ample and smooth polytopes. We next define the projective toric variety $X_A$ determined by a finite set $A$ of lattice points. When $A$ is the lattice points of a polytope $P$ we demonstrate that $X_A$ reflects the properties of $P$ best if $P$ is very ample. We also define the normal fan of $P$ and discuss the relation between the corresponding "abstract" variety $X_P$ and the embedded variety $X_A$.

      

317. ODTU, 13 December 2013, Friday, 15:40
     
     Alexander Degtyarev-[Bilkent] - Viro's patchworking
            

     Abstract:  This is a continuation of my previous talk. After a brief introduction to Hilbert’s 16$^{\rm th}$ problem, I will try to outline the basic ideas underlying Viro’s method of patchworking real algebraic varieties.

      

      

318. Bilkent, 20 December 2013, Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Coordinate ring of a toric variety I
            

     Abstract:    The aim of this talk is to introduce the so called homogeneous coordinate ring of a normal toric variety. We will see how Chow group of Weil divisors turn this ring into a graded ring. Finally we show that every normal toric variety is a categorical quotient.

     
     
     

319. ODTU, 27 December 2013, Friday, 15:40  
     
     Mesut Şahin-[Karatekin] - Coordinate ring of a toric variety II
        

     Abstract:  After the promised example of "bad" quotient, I will review the correspondence between subschemes of a normal toric variety and multigraded ideals of its homogeneous coordinate ring.

2014 Spring Talks

320. Bilkent, 28 February 2014, Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Toric Varieties I 
            

     Abstract:    After recalling briefly basics of sheaf of a divisor on a normal variety, we will concentrate on the toric case. In particular, we give an explicit description of global sections of the sheaf of a torus invariant divisor.

     
     
     

321. ODTU, 7 March 2014, Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Toric Varieties II
             

     Abstract: We will continue with divisors and sheaves on toric varieties. Reference is chapter 4 of Cox, Little and Schenck.

     
     

322. Bilkent, 14 March 2014, Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Toric Varieties III
              

     Abstract:  We will talk about quasicoherent sheaves on the normal toric variety which come from multigraded modules over its Cox ring.

     
     

323. ODTU, 21 March 2014, Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Toric Varieties IV 
            

     Abstract:  We will talk about The Toric Ideal-Variety Correspondence from Cox-Little-Schenck's book Toric Varieties,  see in particular page 220.

     
     

324. Bılkent, 28 March 2014, Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Toric ideal-subscheme correspondence 
         

     Abstract:  .We will talk about the correspondence between closed subschemes in a normal toric variety and B-saturated homogeneous ideals in its Cox ring.

             

325. ODTU, 4 April 2014 Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Multigraded Hilbert functions and toric complete intersection codes 1 
            

     Abstract: We will talk about how multigraded Hilbert functions can be used to compute dimensions of toric codes and list some basic properties of multigraded Hilbert functions. This is a joint work with Ivan Soprunov of Cleveland State University.

      

326. Bilkent, 11 April 2014, Friday, 15:40
     
     Mesut Şahin-[Karatekin] - Multigraded Hilbert functions and toric complete intersection codes 2 
            

     Abstract: We will give a nice formula for the dimension of toric complete intersection codes. We also give a bound on the multigraded regularity of a zero dimensional complete intersection subscheme of a projective simplicial toric variety. The latter is important to eliminate trivial codes. This is a joint work with Ivan Soprunov of Cleveland State University.

      

327. ODTU, 18 April 2014, Friday, 15:40
     
     Emre Coskun-[ODTÜ] - Deformation Theory 1 
            

     Abstract:  In this series of lectures, we will develop deformation theory of functors of Artin rings. After discussing extensions of algebras over a fixed base ring, we will develop the theory of functors of Artin rings. These occur as 'local' versions of various moduli problems, and can give information about the local structure (e.g. smoothness, dimension) of moduli spaces near a point. We apply the theory to concrete examples of moduli problems, such as invertible sheaves on a variety, Hilbert schemes and Quot schemes.

      

      

328. Bilkent, 25 April 2014, Friday, 15:40
     
     Emre Coskun-[ODTÜ] - Deformation Theory 2 
            

     Abstract:   Last week we defined the R-module ExA(R,I). This week we will continue from there and talk about the extensions of schemes.

     
     
     

329. ODTU, 9 May 2014, Friday, 15:40  
     
     Emre Coskun-[ODTÜ] - Deformation Theory 3
        

     Abstract:  This week we will start formal deformation theory. This will be the content of chapter 2 in Sernesi's book.

        
     
       

330. Bilkent, 16 May 2014, Friday, 15:40  
     
     Emre Coskun-[ODTÜ] - Deformation Theory 4
        

     Abstract:  Last time we discussed briefly Schlessinger's theorem. We will continue from there.

          
     
     

331. Bilkent, 21 May 2014, Wednesday, 15:40  
     
     Caner Koca-[Vanderbild] - The Monge-Ampere Equations and Yau's Proof of the Calabi Conjecture
        

     Abstract:  The resolution of Calabi's Conjecture by S.-T. Yau in 1977 is considered to be one of the crowning achievements in mathematics in 20th century. Although the statement of the conjecture is very geometric, Yau's proof involves solving a non-linear second order elliptic PDE known as the complex Monge-Ampere equation. An immediate consequence of the conjecture is the existence of Kähler-Einstein metrics on compact Kähler manifolds with vanishing first Chern class (better known as Calabi-Yau Manifolds). In this expository talk, I will start with the basic definitions and facts from geometry to understand the statement of the conjecture, then I will show how to turn it into a PDE problem, and finally I will highlight the important steps in Yau's proof.

       
     
        
        

332. ODTU, 23 May 2014, Friday, 15:40  
     
     Emre Coskun-[ODTÜ] - Deformation Theory 5
        

     Abstract:  We will discuss the closing remarks of deformation theory for this semester.

          

      

      

333. Bilkent, 27 May 2014, Tuesday, 15:40  
     
     Caner Koca-[Vanderbilt] - Einstein's Equations on Compact Complex Surfaces
        

     Abstract:  After a brief review of Einstein's Equations in General Relativity and Riemannian Geometry, I will talk about one of my results: The only positively curved Hermitian solution to Einstein's Equations (in vacuo) is the Fubini-Study metric on the complex projective plane.

      

      

334. Bilkent, 3 June 2014, Tuesday, 15:40  
     
     Caner Koca-[Vanderbilt] - Extremal Kähler Metrics and Bach-Maxwell Equations
        

     Abstract:  Extremal Kähler metrics are introduced by Calabi in 1982 as part of the quest for finding "canonical" Riemannian metrics on compact complex manifolds. Examples of such metrics include the Kähler-Einstein metrics, or more generally, Kähler metrics with constant scalar curvature. In this talk, I will start with an expository discussion on extremal metrics. Then I will show that, in dimension 4, these metrics satisfy a conformally-invariant version of the classical Einstein-Maxwell equations, known as the Bach-Maxwell equations, and thereby are related to physics (conformal gravity) in a surprising and mysterious way.

2014 Fall Talks

335. Bilkent, 26 September 2014, Friday, 15:40
     
     Alexander Degtyarev-[Bilkent] - Lines on surfaces - I 
            

     Abstract:   This is a joint project with I. Itenberg and S. Sertöz. I will discuss the recent developments in our never ending saga on lines in nonsingular projective quartic surfaces. In 1943, B. Segre proved that such a surface cannot contain more than 64 lines. (The champion, so-called Schur's quartic, has been known since 1882.) Even though a gap was discovered in Segre's proof (Rams, Schütt), the claim is still correct; moreover, it holds over any field of characteristic other than 2 or 3. (In characteristic 3, the right bound seems to be 112.) At the same time, it was conjectured by some people that not any number between 0 and 64 can occur as the number of lines in a quartic. We tried to attack the problem using the theory of K3-surfaces and arithmetic of lattices. Alas, a relatively simple reduction has lead us to an extremely difficult arithmetical problem. Nevertheless, the approach turned out quite fruitful: for the moment, we can show that there are but three quartics with more than 56 lines, the number of lines being 64 (Schur's quartic) or 60 (two others). Furthermore, we can prove that a real quartic cannot contain more than 56 real lines, and we have an example realizing this bound. We can also construct quartics with any number of lines in {0; : : : ; 52; 54; 56; 60; 64}, thus leaving only two values open. Conjecturally, we have a list of all quartics with more than 48 lines. (The threshold 48 is important in view of another theorem by Segre, concerning planar sections.) There are about two dozens of species, all but one 1-parameter family being projectively rigid.

     
     
     

336. ODTU, 2 October 2014, Thursday, 15:40
     
     Alexander Degtyarev-[Bilkent] - Lines on surfaces - II
             

     Abstract:  This is the second part of the previous talk. See the above abstract.

     
     

337. Bilkent, 10 October 2014, Friday, 15:40
     
     Emre Coşkun-[ODTÜ] - Compact Riemann surfaces and algebraic curves-I 
              

     Abstract:  With this talk we start our series of talks on "Girondo and Gonzalez-Diez, Introduction to Compact Riemann Surfaces and Dessins d'Enfants, London Mathematical Society Student Texts 79, Cambridge University Press, 2012." The first chapter is on Riemann surfaces with an emphasis on computable examples.

     
     

338. ODTU, 17 October  2014, Friday, 15:40
     
     Emre Coşkun-[ODTÜ] - Compact Riemann surfaces and algebraic curves-II 
            

     Abstract:  We continue with the topology of  Riemann surfaces.

     
     

339. Bılkent, 24 October 2014, Friday, 15:40
     
     Emre Coşkun-[ODTÜ] - Compact Riemann surfaces and algebraic curves-III 
         

     Abstract:  We will finish the first chapter on compact Riemann surfaces. The main topic this week will be function fields on Riemann surfaces.

             

340. ODTU, 31 October 2014 Friday, 15:40
     
     Özgür Kişisel-[ODTÜ] - Riemann surfaces and discrete groups - I 
            

     Abstract: We will start by discussing the consequences of the Uniformization Theorem of compact Riemann surfaces and continue by discussing the groups which uniformize Riemann surfaces of genus greater than one. Expect lots of pictures.

      

341. Bilkent, 7 November, Friday, 15:40
     
     Özgür Kişisel-[ODTÜ] - Riemann surfaces and discrete groups - II 
            

     Abstract: This week we start with hyperbolic geometry.

      

342. ODTU, 14 November 2014, Friday, 15:40
     
     Özgür Kişisel-[ODTÜ] - Riemann surfaces and discrete groups - III 
            

     Abstract:  We will continue with the fundamental group of compact Riemann surfaces and, time permitting, proceed with the existence of meromorphic functions on such surfaces.

      

      

343. Bilkent, 21 November 2014, Friday, 15:40
     
     Özgür Kişisel-[ODTÜ] - Riemann surfaces and discrete groups - IV 
            

     Abstract:   We will start talking about Fuchsian groups.

     
     
     

344. ODTU, 28 November 2014, Friday, 15:40  
     
     Özgür Kişisel-[ODTÜ] - Riemann surfaces and discrete groups - V
        

     Abstract:  We will talk about automorphisms of Riemann surfaces.

        
     
       

345. Bilkent, 5 December 2014, Friday, 15:40  
     
     Özgür Kişisel-[ODTÜ] - Riemann surfaces and discrete groups - VI
        

     Abstract:  We will talk about the moduli space of compact Riemann surfaces and conclude our discussion of chapter 2.

          
     
     

346. ODTU, 12 December 2014, Wednesday, 15:40  
     
     Sinan Sertöz-[Bilkent] - Belyi's Theorem-I
        

     Abstract: We will describe the content of what is known as Belyi's theorem and prove the hard part which is actually easier than the easy part!

       
     
        
        

347. Bilkent, 19 December 2014, Friday, 15:40  
     
     Sinan Sertöz-[Bilkent] - Belyi's Theorem-II
        

     Abstract: Last week we discussed the content of Belyi's theorem and worked out an example. So it is only this week that we start to prove the first part of Belyi's theorem: If a compact Riemann surface is defined over the field of algebraic numbers, then it has a meromorphic function which ramifies over exactly three points. This is know as the hard part, and the converse is known as the easy part even though the converse is more involved!

          

      

      

348. ODTU, 26 December 2014, Tuesday, 15:40  
     
     Sinan Sertöz-[Bilkent] - Belyi's Theorem-III
        

     Abstract:  This week we will prove that if a compact Riemann surface admits a meromorphic function which ramifies over at most  three points, then it is defined over the field of algebraic numbers. This was first proved by Weil in 1956. We will present a modern proof following Girondo and Gonzalez-Diez.

      

ODTÜ-BİLKENT Algebraic Geometry Seminar 
(See all past talks ordered according to speaker and date)

2015 Spring Talks

349. Bilkent, 13 February 2015, Friday, 15:40
     
     Davide Cesare Veniani-[Leibniz University of Hanover] - Lines on K3 quartic surfaces 
            

     Abstract:  Counting lines on surfaces of fixed degree in projective space is a topic in algebraic geometry with a long history. The fact that on every smooth cubic there are exactly 27 lines, combined in a highly symmetrical way, was already known by 19th century geometers. In 1943 Beniamino Segre stated correctly that the maximum number of lines on a smooth quartic surface over an algebraically closed field of characteristic zero is 64, but his proof was wrong. It has been corrected in 2013 by Slawomir Rams and Matthias Schütt using techniques unknown to Segre, such as the theory of elliptic fibrations. The talk will focus on the generalization of these techniques to quartics admitting isolated ADE singularities.

      

350. ODTÜ, 20 February 2015, Friday, 15:40
          

     Ferruh Özbudak-[ODTÜ] -

     Perfect nonlinear and quadratic maps on finite fields and some connections to finite semifields, algebraic curves and cryptography

     
         
     

     Abstract:    Let K be a finite field with q elements, where q is odd. Let E3 and E2 be extensions of K of index 3 and 2. We show that all perfect nonlinear K-quadratic maps from E3 to E2 are extended affine equivalent (and also CCZ-equivalent). These notions are naturally connected to finite semifields (and to finite projective planes) and to certain important functions in cryptography. The proof is based on Bezout's Theorem of algebraic curves. We also give a related non-extendability result.

         

351. Bilkent, 27 February 2015, Friday, 15:40

     Ali Sinan Sertöz-[Bilkent] - Belyi's Theorem-IV

            

     Abstract:    In the previous talks, the proof of Belyi's theorem was completed modulo a finiteness criterion. In this talk we will prove that  criterion. Namely, we will prove that  a compact Riemann surface S is defined over the algebraic numbers Q¯ if and only if the orbit of S under the action of the Galois group Gal(C/Q) is finite.

         

352. ODTÜ, 6 March 2015, Friday, 15:40

     Davide Cesare Veniani-[Leibniz University of Hanover] - 
     An introduction to elliptic fibrations - part I: Singular Fibres

            

     Abstract:   The theory of elliptic fibrations is an important tool in the study of algebraic and complex surfaces. The talk will focus on Kodaira's classification of possible singular fibres. I will construct some examples of rational and K3 elliptic surfaces to illustrate the theory, coming from pencils of plane cubics and lines on quartic surfaces.  The talk will be aimed at students who took a first course in algebraic geometry.

         

353. Bilkent, 13 March 2015, Friday, 15:40

     Davide Cesare Veniani-[Leibniz University of Hanover] -
     An introduction to elliptic fibrations - part II: Mordell-Weil group and torsion sections

            

     Abstract:    Given an elliptic surface, the set of sections of its fibration forms a group called the Mordell-Weil group. After recalling the main concepts from part I, I will expose the main properties of this group, with a special focus on torsion sections. I will give two constructions on quartic surfaces which appear naturally in the study of the enumerative geometry of lines, where torsion sections play a prominent role.  The talk will be aimed at students who took a first course in algebraic geometry.

         

354. ODTÜ, 20 March 2015, Friday, 15:40

     Ali Sinan Sertöz-[Bilkent] - Belyi's Theorem-V

            

     Abstract:   This is the last talk in our series of talks on Belyi's theorem. In this talk I will outline the proof of the fact  that a compact Riemann surfaceS is defined over the algebraic number field if and only if the orbit of S under the Galois group Gal(C/Q)  contains only finitely many isomorphism classes of Riemann surfaces. Once this is established, we will show that having a Belyi map for S leads to the finiteness of the isomorphism classes in {Sσ}σ∈Gal(C/Q). This will conclude our study of  the first three chapters of Girondo and Gonzalez-Diez's book.

         

355. Bilkent, 27 March 2015, Friday, 15:40

     Ali Sinan Sertöz-[Bilkent] - Exit Belyi, enter dessins d'enfants

            

     Abstract: This week I will first clarify some of the conceptual details of the proof of Belyi's theorem that were left on faith last week. After that we will start talking about dessins d'enfants.

         

356. ODTÜ, 3 April 2015, Friday, 15:40

     Ali Sinan Sertöz-[Bilkent] - From dessins d'enfants to Belyi pairs

            

     Abstract:   We will describe the process of obtaining a Belyi pair starting from a dessin d'enfant.

         

357. Bilkent, 10 April 2015, Friday, 15:40

     Ali Sinan Sertöz-[Bilkent] - Calculating the Belyi function associated to a dessin

            

     Abstract:   I will go over the calculation of  the  Belyi pair corresponding to a particular dessin given in the book, see Example 4.21. Time permitting, I will briefly talk about constructing a dessin from a Belyi pair.

         

358. ODTÜ, 17 April 2015, Friday, 15:40

     Ali Sinan Sertöz-[Bilkent] - From Belyi pairs to dessins

            

     Abstract:   We will talk about obtaining a dessin from a Belyi function.

         

359. Bilkent,  24 April 2015, Friday, 15:40

     Alexander Klyachko-[Bilkent] - Exceptional Belyi coverings

            

     Abstract:   (This is a joint project with Cemile Kürkoğlu.)  Exceptional covering is a connected Belyi coverings uniquely determined by its ramification scheme. Well known examples are cyclic, dihedral, and Chebyshev coverings. We add to this list a new infinite series of rational exceptional coverings together with the respective Belyi functions.  We shortly discuss the minimal field of definition of a rational exceptional covering and show that it is either Q or its quadratic extension.  Existing theories give no upper bound on degree of the field of definition of an exceptional covering of genus 1. It is an open question whether the number of such coverings is finite or infinite.  Maple search for an exceptional covering of g>1  found none of degree 18 or less. Absence of exceptional hyperbolic coverings is a mystery we couldn’t explain.

         

360. ODTÜ, 8 May 2015, Friday, 15:40

     Alexander Degtyarev-[Bilkent] - Dessins d'enfants and topology of algebraic curves

            

     Abstract:   I will give a brief introduction into the very fruitful interplay between Grothendieck's dessins d'enfants, subgroups of the modular group, and topology and geometry of trigonal curves/elliptic surfaces/Lefschetz fibrations.